Cuestion 3 (Amplificador Operacional, convertidor tension corriente)

Cuestion 3 (Amplificador Operacional, convertidor tension corriente)

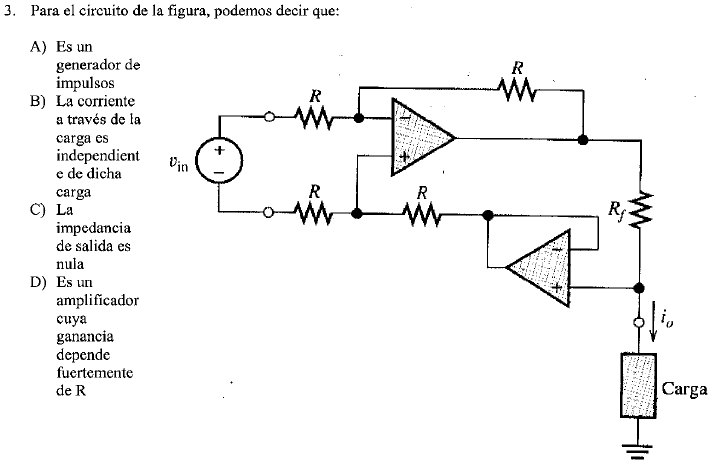
Solucion:

El segundo operacional es un seguidor de tensión con lo que la tensión de salida es la tensión en la carga:

$\begin{displaymath}v_{o_{2}}=v_{L}\end{displaymath}$

Con lo que en el primer operacional tendremos.

$\begin{displaymath}V_{o_{2}}=R\,i+v_{+}\end{displaymath}$

$\begin{displaymath}v_{+}=R\,i-v_{in}+R\,i+v_{-}=2\,R\,i-v_{in}+v_{-}\end{displaymath}$

$\begin{displaymath}v_{+}=v_{-}\end{displaymath}$

$\begin{displaymath}v_{+}=2\,R\,i-v_{in}+v_{-}=2\,R\,i-v_{in}+v_{+}\end{displaymath}$

$\begin{displaymath}0=2\,R\,i-v_{in}\end{displaymath}$

$\begin{displaymath}i=\frac{v_{in}}{2\,R}\end{displaymath}$

$\begin{displaymath}v_{-}=R\,i+v_{o_{1}}\end{displaymath}$

$\begin{displaymath}v_{o_{1}}=R_{f}\,i_{L}+v_{L}\end{displaymath}$

$\begin{displaymath}v_{-}=R\,i+R_{f}\,i_{L}+v_{L}=R\,\frac{v_{in}}{2\,R}+R_{f}\,i_{L}+v_{L}=\frac{v_{in}}{2}+R_{f}\,i_{L}+v_{L}\end{displaymath}$

$\begin{displaymath}v_{-}=v_{+}=V_{o_{2}}-R\,i=v_{o_{2}}-\frac{v_{in}}{2}\end{displaymath}$

$\begin{displaymath}v_{L}-\frac{v_{in}}{2}=\frac{v_{in}}{2}+R_{f}\,i_{L}+v_{L}\end{displaymath}$

$\begin{displaymath}-\frac{v_{in}}{2}=\frac{v_{in}}{2}+R_{f}\,i_{L}\end{displaymath}$

$\begin{displaymath}i_{L}=-\frac{v_{i}}{R_{f}}\end{displaymath}$

Comprobación con el Micro-Cap.

Con lo que respuesta correcta es la b), la corriente en la carga es independiente de la carga.

Siguiente: Cuestion 4 (FET, zona Subir: Examen 2006 Junio 2 Anterior: Cuestion 2 (Diodo, Zener Índice General